LeetCode 141. Linked List Cycle 解題紀錄

2022-02-28 550 words 2 minutes

Contents

題目

Problem

Given head, the head of a linked list, determine if the linked list has a cycle in it.

There is a cycle in a linked list if there is some node in the list that can be reached again by continuously following the next pointer. Internally, pos is used to denote the index of the node that tail’s next pointer is connected to. Note that pos is not passed as a parameter.

Return true if there is a cycle in the linked list. Otherwise, return false.

Example 1:

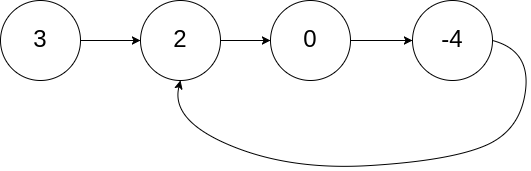
Input: head = [3,2,0,-4], pos = 1
Output: true

Explanation: There is a cycle in the linked list, where the tail connects to the 1st node (0-indexed).

Example 2:

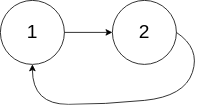
Input: head = [1,2], pos = 0
Output: true

Explanation: There is a cycle in the linked list, where the tail connects to the 0th node.

Example 3:

Input: head = [1], pos = -1
Output: false

Explanation: There is no cycle in the linked list.

Constraints:

The number of the nodes in the list is in the range $[0, 10^4]$.
$-10^5 \leq$ Node.val $\leq 10^5$ pos is -1 or a valid index in the linked-list.

Follow up: Can you solve it using $\mathcal{O}(1)$ (i.e. constant) memory?

想法

這題我看了 n 遍才看懂他在幹嘛 orz

新增兩個指標，分別叫做 walk 和 run ，每一秒走一個、跑兩格。

前三個點為單向通行，不在 cycle 裡面，後五個點屬於 cycle 之中。

如果這個 linked list 存在 cycle，則 walk 和 run 總有一秒會相遇。

下圖中可以看到：

第零秒兩個同時從 1 出發，到第五秒時兩個在 6 相遇。

相遇時間

至於要花幾秒才會相遇呢?

先耗費走完 line 的時間
當 walk 進入 cycle 中後，再來只要看 cycle
line % cycle 是在等待 walk 進入 cycle 時，run 的位置
run 應花費 cycle - (line % cycle) 的時間追上 walk
設 $line = 3$ 和 $cycle = 5$
$ line+(cycle-(line\mod cycle))$
$= 3 + (5-(3 \mod 5)) $
$= 3+(5-3) $
$= 3+2 = 5 $
⇒ 總共需要 $5 秒/steps$

pos 就是這樣反推出來ㄉ凸^-^凸

解法

bool hasCycle(ListNode *head) {

    if(!head)   return head;

    // walk 和 slow 都從起點出發
    ListNode *walk = head, *run = head;

    while(run->next && run->next->next)
    {
        // 一次走一步
        walk = walk->next;

        // 一次走兩步
        run = run->next->next;

        // walk 和 run 會重疊代表著 linked list 中有迴圈
        if(walk == run)
        {
            return 1;
        }
    }

    return 0;
}

Time complexity: $\mathcal{O}(n)$.
Space complexity: $\mathcal{O}(1)$.